1.  凸多边形的外角和等于 ${360}^{\circ }$.

规范场理论(Gauge Theory)

 

参考资料

D. S. Freed, K. K. Uhlenbeck:  Instantons and four-Manifolds.

H. B. Lawson, Jr.:  The theory of gauge fields in four-dimension.

K. Donaldson & Kronheimer:  Geometry of Four-Manifolds.

 

 

几何参考书

K. Kobayashi, K. Nomizu,  Foundations of Differential Geometry.

J. Milnor, J. Stasheff,  Characteristic classes.

N. Steenord, The topology of Fibre Bundles.

Aubin, Nonlinear Problems in Riemann manifolds.

Atiya,  K-theory.

 

[分析]

 

Y:  Sunrise Time (LST)
Y2=X2-W2*4/1440

Z:  Sunset Time (LST)
Z2=X2+W2*4/1440

X:  Solar Noon (LST)
X2=(720-4*$b$4-V2+$B$5*60)/1440

这里 $B$4 :  longitude,   $B$5:  timezone

 

V:  Equation of time (时差)  单位: minutes

V2=4*DEGREES(U2*SIN(2*RADIANS(I2))-2*K2*SIN(RADIANS(J2))+4*K2*U2*SIN(RADIANS(J2))*COS(2*RADIANS(I2))-0.5*U2*SIN(4*RADIANS(I2))-1.25*K2*K2*SIN(2*RADIANS(J2)))

 

即,

$$\begin{array}{rl}V2& =4\cdot [U2\cdot \sin (2\cdot I2)-2\cdot K2\cdot \sin (J2)+4\cdot K2\cdot U2\cdot \sin (J2)\cdot \cos (2\cdot I2)\\ & -\frac{1}{2}\cdot U2\cdot \sin (4\cdot I2)-\frac{5}{4}\cdot K2\cdot K2\cdot \sin (2\cdot J2)]\end{array}$$

 

I.  (Geom Mean Long Sun)  (deg)

I2=MOD(280.46646+G2*(36000.76983+G2*0.0003032),360)

 

J.  (Geom Mean Anom Sun)  (deg)

J2=357.52911+G2*(35999.05029-0.0001537*G2)

 

G.  Julian Century

G2=(F2-2451545)/36525

这里 2451545 对应到 2000年1月1日.

---

F.  Julian Day(儒略日)

$$F2=D2+2415018.5+E2-\$B\$5/24$$

$B$5 是 Time Zone (+ to E), E指 East.

 

D, Date,  D2=$B$7,  如: 2010/6/21

 

Julian Day

For example: The Julian day number for the day starting at 12:00 UT on January 1, 2000 was 2,451,545.

 

E.  Time (past local midnight). 从午夜开始经过的时间

$$E(i+1)=E(i)+0.1/24,E(2)=0.1/24$$

240行, 24h/240=0.1h=6min

所以

$$\begin{array}{rl}F2& =D2+2415018.5+E2-\mathrm{\$}B\mathrm{\$}5/24\\ & =2010/6/21+2415018.5+0:06:00-1/24\\ & =2455368.75\end{array}$$

这推出 $2010/6/21+0:06:00\approx 40350.29166667$.

 

 

儒略日(Julian Day) 的起点定在公元前 4713 年(天文学上记为 $-4712$ 年)1月1日格林威治时间平午(世界时间12:00). 即 JD 0 指定为 4713 B.C. 1月1日 12:00 UT 到 4713 B.C. 1月2日 12:00 UT 的 24 小时. 每一天赋予了一个唯一的数字, 顺数而下.

如: 1996年1月1日12:00:00的儒略日是 2450084

     1899年12月29日  --- 2415018

这个日期是考虑了太阳、月亮的运行周期, 以及当时税收的间隔而定下来的.

Joseph Scliger 定义儒略周期为 7980年.  $7980=\text{l.c.m.}(19,15,28)$.

---

 

28年为一太阳周期(Solar cycle). 经过一太阳周期, 则星期的日序与月的日序会重复.

19年为一太阴周期, 或称默冬章(Metonic cycle), 因235朔望月=19回归年, 经过一太阴周期则阴历月年的日序重复.

15年为一小纪(indiction cycle), 此为罗马皇帝君士坦丁(Constantine)所颁, 每15年评定财产价值以供课税, 成为古罗马用的一个纪元单位.

故以7980年为一儒略周期, 而所选的起点公元前4713年, 则是这三个循环周期同时开始的最近年份.

 

注: 7980 是这三个数的最小公倍数.

>> lcm(28,19,15)
in> lcm(28,19,15)
7980

 

---

儒略日(Julian Date)的计算

$$\begin{array}{rl}a& =[(14-\text{month})/12]\\ y& =\text{year}+4800-a\\ m& =\text{month}+12a-3\end{array}$$

则格里历日期的中午时候

$$\text{JDN}=\text{day}+[(153m+2)/5]+365y+[y/4]-[y/100]+[y/400]-32045,$$

若日期为儒略历, 则

$$\text{JDN}=\text{day}+[(153m+2)/5]+365y+[y/4]-32083.$$

儒略日的简化

$$\text{MJD}=\text{JD}-2400000.5$$

---

 

U
(var y)
U2=TAN(RADIANS(R2/2))*TAN(RADIANS(R2/2))

即, $U2={\tan }^2\frac{R_2}{2}$.

 

R  (Obliq Corr)  (deg)

R2=Q2+0.00256*COS(RADIANS(125.04-1934.136*G2))

即

$$R_2=Q_2+0.00256\cdot \cos (125.04-1934.136\cdot G_2)$$

 

 

Q.  (Mean Obliq Ecliptic)   (deg)

Q2=23+(26+((21.448-G2*(46.815+G2*(0.00059-G2*0.001813))))/60)/60

 

K.  Eccent Earth Orbit   (eccentricity of earth)   地球的离心率

K2=0.016708634-G2*(0.000042037+0.0000001267*G2)

 

 

---

[待修改]

 

 

References:

https://blog.csdn.net/caolaosanahnu/article/details/7890008

Julian Day Number Calculations (numerical.recipes)

 

$T\in D_n(U)$ 称为一个 rectifiable current, 如果对所有的 $\omega \in D^n(U)$, 有

$$T(\omega )={\int }_M⟨\omega (x),\xi (x)⟩\theta (x)\mathrm{d}{H}^n(x)$$

这里 $M$ 是 $U$ 中的 $H^n$-可测的可数 $n$-rectifiable 子集.

$\theta$ 是局部 $H^n$-可积的正函数  (叫做重数函数).

$\xi :M\to {\mathrm{\Lambda }}_n{\mathbb{R}}^p$ 是一个 $H^n$-可测函数, 满足下面的关系: 在几乎处处 $H^n$-可测的点 $x\in H$ 处(at $H^n$-a.e. $x\in H$), 有 $\xi (x)={\tau }_1\wedge \cdots \wedge {\tau }_n$, 其中 $\{{\tau }_1,\dots ,{\tau }_n\}$ 是 $T_{x}M$ 的一个标准正交基.

记 $T=\tau (M,\theta ,\xi )$.

若 $\theta$ 是取整数值的, 则这样的 current 被称为是一个整数可乘性的 rectifiable current. (an integer multiplicity rectifiable current)

 

定理. 若 $V\subset U\subset {\mathbb{R}}^{n+1}$ 具有局部有限的周边(locally finite perimeter), 即 ${\chi }_V\in {\mathrm{BV}}_{loc}(U)$, 则 $\partial [[V]]$ 是一个 integer multiplicity current, 满足 $\theta (x)=1$ 对 $H^n-$ a.e. $x\in M$.

 

 

 

---

Reference:

来源于笔记

 

 

 

 

 

 

 

 

 

 

 

 

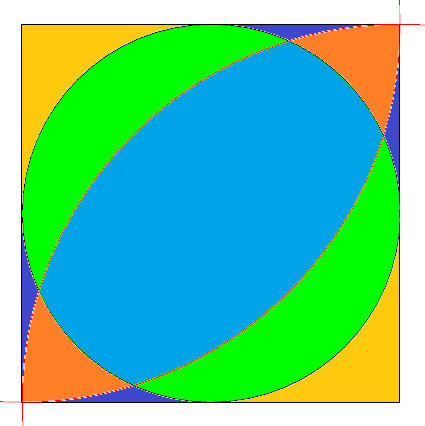
假设正方形的边长是 10, 求图中绿色部分的面积.

 

 

Answer:  单个的绿色部分面积为

$$25\arccos \frac{1}{2\sqrt{2}}-100\arccos \frac{5}{4\sqrt{2}}+\frac{25}{2}\sqrt{7}$$

 

---

题目来源: Lei Liu (刘磊)

https://marcosnietoblog.wordpress.com/2014/02/22/source-code-inverse-perspective-mapping-c-opencv/

计算机视觉

证明.

$$\text{Vol}(S^{2n-1})=\frac{2{\pi }^n}{(n-1)!}$$

$$\text{Vol}(S^{2n})=\frac{2^{n+1}{\pi }^n}{(2n-1)!!}$$

---

注意.

$S^{2n-1}$ 的球坐标为

$$\{\begin{array}{ll}{x}_1& =\cos {\theta }_1\\ x_2& =\sin {\theta }_1\cos {\theta }_2\\ x_3& =\sin {\theta }_1\sin {\theta }_2\cos {\theta }_3\\ & ⋮\\ x_{2n-1}& =\sin {\theta }_1\sin {\theta }_2\cdots \sin {\theta }_{2n-2}\cos {\theta }_{2n-1}\\ x_{2n}& =\sin {\theta }_1\sin {\theta }_2\cdots \sin {\theta }_{2n-2}\sin {\theta }_{2n-1}\end{array}$$

 

$S^{2n}$ 的球坐标为

$$\{\begin{array}{ll}{x}_1& =\cos {\theta }_1\\ x_2& =\sin {\theta }_1\cos {\theta }_2\\ x_3& =\sin {\theta }_1\sin {\theta }_2\cos {\theta }_3\\ & ⋮\\ x_{2n}& =\sin {\theta }_1\sin {\theta }_2\cdots \sin {\theta }_{2n-1}\cos {\theta }_{2n}\\ x_{2n+1}& =\sin {\theta }_1\sin {\theta }_2\cdots \sin {\theta }_{2n-1}\sin {\theta }_{2n}\end{array}$$